REACTIONS DETERMINATION IN DETERMINATE STRUCTURE...PART TWO

-

 

Reactions as what support offers to restrain structure to its position, when subjected to loadings.

As the degree of indeterminacy of determinate structure n= 0, this means the reactions or forces are balancing each other with no extra reaction (structure is in equilibrium).

Due to balancing reactions, the reactions can easily be determined by using three equilibrium equations for the plane structure, which are the following






The magnitude and direction of support reactions in determinate structure can easily determined by adhering the following procedures

·         Drawing free body diagram FBD

·         Check for determinacy

·         Applying equilibrium equations


Example 1

Determine support reactions of the structure below



Solution

The first step is to draw free body diagram, this diagram show the directions (proposed) and position of forces and reactions, so they can easily analyzed.

The support A provide two reactions Ax   and Ay as at point A we have fixed support, and at point B we have only one reaction as we have roller support.

The direction arrows should be indicated as the directional reference to your analysis.


Second step is to check determinacy, this step help to know if the structure is statically determinate so we can analyze it by three equilibrium equation or not, unstable structures will not be analyzed as they cannot support desired loadings.

From degree of indeterminacy, n = r – 3

                                                         r = 3

                                                    n = 0 (the structure is statically determinate)

Step 3 Since it is statically determinate, then reactions can be determined by three equilibrium equations


 


             







Example 2

Determine the support reaction of the structure below carrying both point load inclined at 600 and distributed load.


Solution

The first step is to draw free body diagram, this diagram show the direction (proposed) and position of forces and reactions, so they can easily analyzed.

The support A provide only one reaction RA as at point A we have roller support, and at point C we have two reactions Cx and Cy as we have pin support.

But reaction RA at support A will have two directional effects as Ax and Ay because the reaction is inclined.

The direction arrows should be indicated as the directional reference to your analysis.


Second step is to check determinacy, this step help to know if the structure is statically determinate so we can analyze it by three equilibrium equation or not, unstable structures will not be analyzed as they cannot support desired loadings.

From degree of indeterminacy, n = r – 3

                                                         r = 3

                                                    n = 0 (the structure is statically determinate)

Step 3 Since it is statically determinate, then reactions can be determined by three equilibrium equations.

We need to resolve the inclined force into horizontal and vertical components for easy calculation in equilibrium equation.

Hz = 10cos 600

      = 5KN

Vt  =   10sin 600

      = 8.66KN














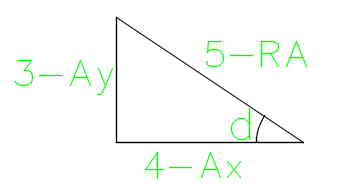
Comments

Post a Comment

Popular posts from this blog

HOW TO FIND THE MEAN GIRTH OF THE SIMPLE BUILDING

-
Image
Mean girth of building , this is the centerline perimeter or circumference of the building, which describes length of the building. The following text show how to find the mean girth of simple building plan shown below for superstructure walling which can help in quantity determination of different materials to be applied for paint work, plaster work, blocks or brick work. Important Terms 1. External Dimensions.   These are dimensions measured from      external surface of the building's walls, these always show the extent of building on plot. 2. Internal Dimensions. These are dimensions measured from internal surfaces of the building walls, these always show sizes of rooms. 3. Corner. These are interconnection or points where two non colinear walls meet. 4. Recesses. There are the partition or adjoining walls which forms rooms or which were not considered in exterior girth of building determination. Ti...
Read more

HOW TO CALCULATE THE NUMBER OF BLOCKS REQUIRED FOR WALLING

-
Image
The number of blocks required for wall can be calculated from the area of the wall which will be constructed by the block of certain size. The mean girth of the simple obtained as calculated from previous post is 28.85m The common (standard) size of the conc. block is 150 x 230 x 450; the wall length (mean girth) of building is multiplied by the height of the walls to find the total  surface area of wall, for a wall of height 2.8m with the opening as shown in the table below The table below shows windows and doors schedule for doors and windows used   Schedule for windows used PORTIONS WIDTH(m) HEIGHT(m) NUMBER(m) AREA(m 2 ) A 1.50 1.50 3 6.75 B 1.50 1.50 2 4.50 C 0.7 0.7 1 0.49 1.00 1.50 1 1.50 D 1.50 1.50 1 2.25 1.00 1.50 1 1.50 Total Area of All Wind...
Read more

HOW TO CALCULATE AMOUNT OF MORTAR PER S.Q.M FOR BLOCK WALL

-
Image
HOW TO CALCULATE AMOUNT OF  MORTAR  PER S.Q.M FOR BLOCK WALL The amount of cement paste required as mortar per square meter can be calculated through the following steps 1.       Knowing the dimension of the blocks to be used for construction. Here, let’s say we use the cement concrete block of size 150 x230 x450 where by 150 s the thickness of the wall. These dimension s (all dimension are in mm) help to find the area of one block forming wall surface as follows Area of one block = 230 x 450                              = 103,500mm 2 2.        Knowing the new dimension of blocks with cement mortar. New dimension of concrete block means the height and length of a block with the thickness of mortar which is 15m, then the new height id 245mm and new length is 265mm Fr...
Read more